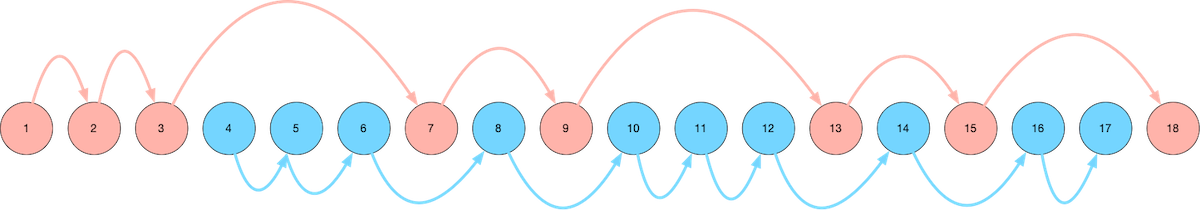
A visual map of our route through Ulysses. Read on for an explanation...

A Little Bit About Ulysses

Let's talk about Ulysses for a minute.

Ulysses is regarded as one of the greatest books ever written. But, of course, it's also barely comprehensible when you first read it.

Getting to know why Ulysses is so famous requires a lot of context, in the same way that understanding what makes a film such a classic requires not just an appreciation of the aesthetics and the experience of the film, but also the historical, social, and cultural context, the story surrounding the story.

The best way we can describe Ulysses is saying that it is a meta-novel. It is a novel that is nominally about June 16, 1904, and how the two main characters spend that day - but throughout the story, there are so many more layers that are added on - linguistics, art, language, history all make their appearances (as does just about every major bodily function).

The novel is meta in the way that it weaves together layer upon layer of storytelling and narrative tehnique, aping so many different styles (newspaper headings, Old English legends, Irish mythology, vignettes that have the feel of a short indie film, even FAQs).

How We Read Ulysses

Our first time reading through Ulysses was in 2011, (ironically) as a way of taking a mental break from the stress of school. We had a special affinity for Joyce based on reading his short story collection Dubliners, so making it through Ulysses was a goal we had had for a while.

Almost immediately, it was clear that this was not a book that could simple be "read", start to finish, the way a normal book is read. No, Ulysses had more in common with a physics textbook than it did with your typical novel. It is a thing to be experienced and studied, and taken slowly.

We spent the first three chapters of Ulysses extremely confused and without any hope of finishing the novel. But then, we discovered two game-changing strategies:

First, we consulted books about Ulysses. Visit any academic library and you'll likely find an entire shelf of books on Ulysses, but there are a few that are considered classics. One of the works that is considered a definitive interpretation of Ulysses was written by Stuart Gilbert, who published his commentary on Ulysses in 1930. Gilbert knew Joyce personally, lending an air of officiality to the interpretation. The commentary includes a chapter-by-chapter discussion of themes, images, symbols, callbacks to Homer's Odyssey, and key quotes to help make certain passages of Ulysses stand out in the mind much more clearly. This book gave us the road map to each chapter of Ulysses, and crucially, helped us realize that the chapters don't need to be read in linear order - there are many converging and diverging timelines in Ulysses and there are many routes through the book.

Second, we discovered a wonderful audiobook version of Ulysses from Naxos audiobooks. (We started with Librivox recordings, which were very hit-and-miss. Professionally-produced audiobooks are a cut above, and well worth seeking out.)

When we discovered audiobooks, we sometimes listened to the audio book version of the Ulysses chapter by itself, and sometimes we read along with the audio recording. Either way, it's a very different way to experience a book, to hear it read aloud, and doubly so with Ulysses, which has many sections that seem to take on a completely different tone and life when they are read aloud versus read off of the page.

Two Parallel Routes Through Ulysses

As we got to know Ulysses better, we gravitated toward Leopold Bloom's character. In particular, we charted two different routes through the book - the first (mostly) following Leopold Bloom, and the second following Stephen Dedalus. Here's a visual guide to our map to reading Ulysses:

We start with the blue route, which means the first few chapters of Ulysses that we read are Chapters 4, 5, and 6. This is out of order, but the important thing is enjoying the experience of reading Ulysses - and Chapters 4, 5, and 6 are far more enjoyable and comprehensible than Chapters 1, 2, and 3.

The Blue Route

The Blue Route through Ulysses (the first route that we take when we read it) consists of the following chapters:

• Chapter 4 - Calypso
• Chapter 5 - Lotus Eaters
• Chapter 6 - Hades
• Chapter 8 - Lestrygonians
• Chapter 10 - Wandering Rocks
• Chapter 11 - Sirens
• Chapter 12 - Cyclops
• Chapter 14 - Oxen of the Sun
• Chapter 16 - Eumaeus
• Chapter 17 - Ithaca

This route follows Bloom from the start of his day (Chapter 4), follows him as he wanders about town in the morning (Chapter 5), attends a funeral (Chapter 6), eats lunch (Chapter 8), visits a pub in the afternoon (Chapter 12), and visits a hospital in the evening (Chapter 14).

The two main characters, Stephen Dedalus and Leopold Bloom, finally meet in Chapter 14, so Chapters 16 and 17 cover the drunken evening and long path back home that the two take.

Chapter 17 is an absolute delight and takes the cake as our favorite chapter. It takes the form of an FAQ, and reads almost like a technical specification for some omnipotent entity that will one day need to reproduce, from scratch, every detail of the scenes depicted in Chapter 17.

Taken on its own, the Blue Path may leave out "notable" or "significant" chapters of Ulysses, but the selection is lighter and more enjoyable than reading the novel start to finish.

One curious aspect of the Blue Path (perhaps true of the novel in general, though) is the way the chapters seem to balloon in size, and sometimes in complexity. For example, Chapters 4, 5, 6, and 8 are similar in style, yet Chapter 4 is far shorter than Chapter 8; by the time the novel reaches Chapter 8 the narrative begins to wander a great deal, the stream of consciousness grows thicker and more complicated with each page, the narrative captures ever more goings-on, and time seems to stretch and dilate as the novel progresses.

(The novel's longest chapter, Chapter 15, comes near the end of the Red Path.)

Regarding the way the Blue and Red Paths read the novel out of order - one of the things that makes it so easy to read Ulysses out of order is the nebulous nature of cross-references in the book. You could read the entire novel backwards and probably find the same structure of references as if you were reading it forward. Chapter 8 references Chaper 14, Chpater 12 references Chapter 8, then 14, then 11, 5 references 3, 14 references everyone, and so on. Certain themes, words, motifs, and images recur through the book, like cycles.

Ulysses must be pieced together, and read many times. Whatever order you read it in, reading the text is like scouring for clues in a crime scene, except it's a perfectly-preserved crime scene that yields infinite clues. The deeper you dig, the more clues you find, the more intricate the weaved web becomes.

The Blue Path makes Ulysses lighter, easier, and more digestible. If you only finish the Blue Route, it's an accomplishment all in itself!

The Red Route

The Red Route through Ulysses (the second route that we take through the book) consists of the following chapters:

• Chapter 1 - Telemachus
• Chapter 2 - Nestor
• Chapter 3 - Proteus
• Chapter 7 - Aeolus
• Chapter 9 - Scylla and Charybdis
• Chapter 13 - Nausicaa
• Chapter 15 - Circe
• Chapter 18 - Penelope

The Red Route through the novel mainly follows Stephen Dedalus (with the exception of Chapter 7 (Aeolus), but it links up with Chapter 9 (Scylla and Charybdis)). Be warned, the Red Route chapters start off easy with Chapters 1 and 2, but Chapter 3 (Proteus) feels like intellectual quicksand, and is a significant hurdle to getting through the novel (hence its inclusion in the Red Path).

Chapter 3 is difficult because it's a rambling stream of consciousness from Stephen Dedalus, who is a lot more intellectual (professorial) and abstract than Leopold Bloom. It makes Chapter 3 one of the more difficult chapters in Ulysses. But after taking the entire Blue Path through the novel, Chapter 3 is easier to handle - the stream of consciousness format is familiar, but more abstract than the streams of consciousness we saw from Leopold Bloom. Being familiar with the stream of consciousness format makes it easier to wade through the generous heaping of historical, literary, and linguistic references in Chapter 3.

(Side note: Chapter 3 is when we first discovered the wonder of asking the library for help understanding what the hell is going on in Ulysses. It turns out, a lot of people have a lot to say, and the last thing anyone should do is get frustrated trying to make it through Chapter 3 by themselves!)

Chapter 7 (Aeolus) is left out of the Blue Path because its format of newspaper-like headlines and paragraphs is a significant break from the stream of consciousness format of Chapters 4, 5, 6, and 8.

Chapter 9 is like the scene in the movie when we think the two storylines are finally going to converge, except it turns out to be a near-miss, and the storylines remain separate. Bloom visits the library to retrieve a picture of two keys for an advertisement he's placing in the newspaper (for a client) in Chapter 7, while Stephen is holding forth on Shakespeare in a discussion at the same library.

Chapter 13 is the infamous chapter that got Ulysses banned for its stream of consciousness description of Bloom getting his jollies off while watching fireworks at the beach. Another deviation of the Red Path back to Bloom, but we think Chapter 13 is better appreciated after going through the Blue Path - especially after Chapter 14.

Chapter 15 is, hands down, the single most difficult chapter of Ulysses, but also the richest. Chapter 15 is best experienced by not reading it start-to-finish, not reading it off the page, but by hearing it, by visualizing it. The less you read Chapter 15 like a novel, the more you get out of it.

Chapter 18 is the perfect way to end the book. Reading Chapter 18 at the end of the Red Path is like finishing a long, excruciating, multi-day excursion through the woods by sitting beside a babbling brook, peeling off your socks, and sticking your feet in the stream to soak for an hour or two.

And that's it - that's my recommended path through Ulysses in a nutshell.

More Resources

Notes on Ulysses on our charlesreid1.com wiki: https://charlesreid1.com/wiki/Ulysses

Notes from our (very first) 2011 reading of Ulysses: https://charlesreid1.com/wiki/Ulysses/2011

Notes from our 2016 reading of Ulysses (the first year we charted the Blue Path and the Red Path): https://charlesreid1.com/wiki/Ulysses/2016

Notes from our (current) 2022 reading of Ulysses: https://charlesreid1.com/wiki/Ulysses/2022

Happy 100th Birthday, Ulysses!

A very happy (belated) 100th birthday to one of the greatest novels of all time, if only measuring by the enjoyment that we have extracted from this novel-puzzle-enigma-slash-undefinable-book-thing.

Ulysses was first published on February 2, 1922, a little over 100 years ago today.

We think the Wikipedia article for Ulysses sums it up best:

The publication history of Ulysses is complex.

• https://en.wikipedia.org/wiki/Ulysses_(novel)#Publication_history

This post is part 5 of a series.

What is a Zettelkasten?

See Part 1 of this series for a little more background on what we're talking about! But basically:

• A zettelkasten is a system of note-taking, where notes are kept simple and tags, or categories, are added to notes to interlink them and create a network of connected notes that aid in discovery and memory.

• MediaWiki (the software that runs Wikipedia) is a mature PHP software package for creating a wiki, that has many built-in features that make it very amenable for use as a zettelkasten.

Todo Lists

This post will use information covered in our previous posts, mainly from Part 3 on organizing pages, but applied to pages in the wiki that are dedicated to keeping track of todo lists.

The Todo Category

Before we get into how we organize todo lists, we should mention that we make heavy use of this pattern in our wiki, so we have many todo lists. To keep track of all the todo lists, it can be useful to have a way to get a list of todo lists.

Whenever we create a new todo list page, we always add the category [[Category:Todo]] to the todo list. If we need to see all our todo lists in one place, we just visit that Category:Todo page, and MediaWiki will automatically generate a list of all todo lists.

Start with Top Level Sections

When starting a todo list page, we use a strategy covered in Part 3, which is to reserve top-level sections for the different purposes the page may serve.

Typical top-level sections in our todo list pages are:

• Overview - used if we are revisiting the page and need a brief summary of the most important information
• Plan - contains planned todo items, work that is not yet in progress
• Todo - contains items that are currently in progress, usually notes from today or this week (and empty if a todo page is not being actively used)
• Done - items that were previously in Todo, but were completed
• Notes - a top-level section that contains notes about the todo list topic. Not necessarily todo items, but useful/related to the todo list.

The Notes section is useful when the todo list is still taking shape; the Overview section is useful when the todo list is done, and there is a concrete outcome or important summary information to refer back to. The Plan section is useful as a catch-all basket for future tasks, or tasks that aren't being addressed yet. And so on.

Topic Subsubsections

The top-level sections of the page are dedicated to different uses of the page: Overview, Planned Todo Items, In-Progress Todo Items, Completed Todo Items, Notes, etc.

Within those sections, the second-level headers organize information and notes by date. The second-level headers have the form YYYYMMDD.

The third-level headers are topic headers. If a single todo item requires completing tasks A, B, and C, which are completed on the same date, then tasks A, B, and C would have their own third-level headers.

As a more concrete example, if I start working on a task to "Foo the bar" on 2016-01-01, I will go to the top-level section for In-Progress Todo Items, add a subsection 20160101, and then add a sub-subsection "Foo the bar":

=Todo In Progress=

==20160101==

===Foo the bar===

Lorem ipsum dolor sit amet, consectetur adipiscing elit. Praesent maximus, purus a gravida suscipit, urna neque
pharetra sem, in luctus nisl arcu eu risus. Aliquam vulputate ac tortor et ornare.

Todo Subpages

What if halfway through task C, things start to get complicated? Starting notes at a third-level header doesn't leave room for many more headers - so if you find yourself needing to organize notes for task C into their own sections and subsections, start a subpage. If your todo list is at [[Todo/Foobar]], then split the notes for task C into their own subpage, [[Todo/Foobar/Task C]]. Create links between the two pages, so it's easier to navigate to/from them.

Notes First, Overview Last

In terms of order, we usually organize the top level sections of the page in the order we've specified above: Overview, Planned Todo Items, In-Progress Todo Items, Done Todo Items, and Notes. These can be modified to suit your own purposes or the particular todo list.

Usually, we will start with the last section - Notes - and end with the first section - the Overview.

The Notes section is where we start when we're trying to figure out how to organize the todo list page. The notes section is typically only useful when you're starting up a todo list, which is why it goes at the bottom - it is rarely used once the todo list is started.

The Overview section is where we summarize important outcomes from various todo tasks. It might be a summary of major efforts, or a list or table that's the outcome of all of the work on the todo list. In any case, if you need to add an Overview section, you'll know it.

An Example

We have an example page showing this pattern in action. It's here: https://charlesreid1.com/wiki/Todo/Foo

More Details

For more details, see the full writeup on our (public non-zettelkasten!) wiki here: https://charlesreid1.com/wiki/Zettelkasten/Patterns/Todo_Lists

This post is part 4 of a series.

What is a Zettelkasten?

See Part 1 of this series for a little more background on what we're talking about! But basically:

• A zettelkasten is a system of note-taking, where notes are kept simple and tags, or categories, are added to notes to interlink them and create a network of connected notes that aid in discovery and memory.

• MediaWiki (the software that runs Wikipedia) is a mature PHP software package for creating a wiki, that has many built-in features that make it very amenable for use as a zettelkasten.

Organizing a Page

The way that pages are organized on a wiki is central to the way the entire wiki is structured. While it may seem like a trivial topic that anyone can figure out as they go, a zettelkasten is intended to hold notes accumulated over many years, and as pages accumulate more information they can start to become inconsistent, crowded, and messy.

We are here with some battle-tested patterns to help manage that mess and keep pages organized and useful (for all of the many purposes a note in a zettelkasten may need to serve) for years to come.

The three patterns we use to help organize pages on the MediaWiki zettelkasten are:

1. Reserve top-level headers for meta-level page organization (different sections for different uses of the page).
2. Take notes chronologically in a top-level Notes section.
3. Move information to sub-pages as it accumulates or if requires more room.

What Problem Are We Trying To Solve?

To understand what problem these patterns are trying to solve, suppose you have your MediaWiki zettelkasten, and you start a single note that is on a broad topic. The note might start with a simple structure, have its own internal logic, and be organized consistently. Easy as that!

But now suppose you come back to that same topic three months later, with a different perspective and a different purpose. Maybe you're starting a new project, and re-using some information from the existing note but also adding new information. Or maybe you learned some new information that changes the way the page should be organized.

With a page in place, with its own structure and organization and logic, it can be difficult to know where to incorporate new information down the road. The ideal note-taking system eliminates that kind of internal friction, and makes it as easy as possible to capture new information, without the cognitive burden of having to reorganize the page again and again.

Pattern 1: Reserve Top Level Sections

This strategy is a small and easy-to-implement change in how new pages are created, that keeps the page flexible for multiple different uses.

The strategy is this: when creating a page, the initial version of the page should only use second-level or deeper section headers, so any section like =Specifications= would become ==Specifications==.

Yup, that's it! We also have a simple example of a MediaWiki page before/after applying this pattern here: https://charlesreid1.com/wiki/Zettelkasten/Patterns/Page_Organization#Pattern_1:_Reserve_Top_Level_Sections

Pattern 2: Taking Notes Chronologically

We reserve top-level headers for dividing the page based on its different uses. If we want to start taking notes, we can add a top-level Notes section to the bottom and start taking notes there.

To keep notes organized chronologically, we add a second-level header with the year, month, and date. That way, while we're taking notes, we don't have to worry about how to incorporate the information into the existing "Overview" information, or try and filter the information as we go. We add notes to the notes section, and can copy or move information to other parts of the page later.

Here is a simple example of a MediaWiki page after applying this pattern: https://charlesreid1.com/wiki/Zettelkasten/Patterns/Page_Organization#Pattern_2:_Taking_Notes_Chronologically

Pattern 3: Subpages

While following the pattern covered above, you might find that on a particular day, working on a particular task, the notes for that task end up being much more complicated than initially expected - it might involve research, links, tasks, notes, and in general require more room than the sub-sub-subsection allotted to it by using the two patterns we have covered.

In that situation, you can replace the sub-sub-section's text with a link to a subpage (for example, on the [[Foobar]] page, if you are working on Baz and it gets complicated, you create a subpage [[Foobar/Baz]]). The original page should link to the subpage, and vice-versa, to make it easy to navigate. Any relevant Category tags should also be added to the subpage, to help make it findable.

This page shows another simple MediaWiki example page showing the subpage pattern in action: https://charlesreid1.com/wiki/Zettelkasten/Patterns/Page_Organization#Pattern_3:_Subpages

More Details

For more details, see the full writeup on our (public non-zettelkasten!) wiki here: https://charlesreid1.com/wiki/Zettelkasten/Patterns/Page_Organization